Handwritten notes, page 1/5 •
[previous page] •
[next page] •
[PDF]
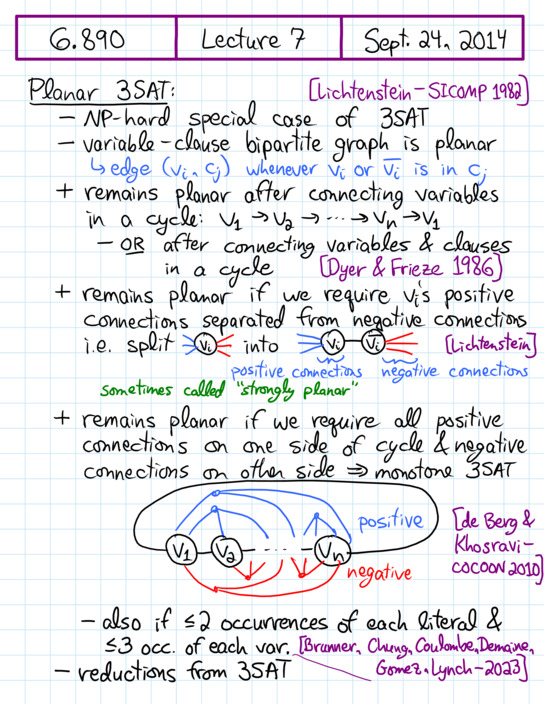
Handwritten notes, page 1/5 • [previous page] • [next page] • [PDF]
Slides, page 1/37 •
[previous page] •
[next page] •
[PDF]
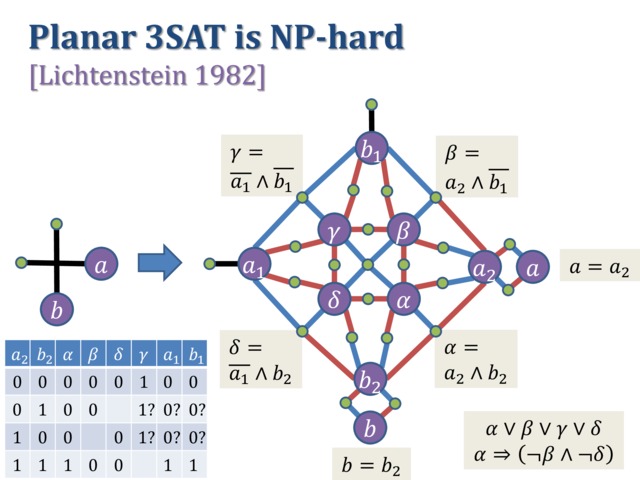
Figure drawn by Erik Demaine based on Figure 4 of http://dx.doi.org/10.1137/0211025
Slides, page 1/37 • [previous page] • [next page] • [PDF]