|
[+]
3SUM & APSP. k-SUM upper and lower bounds, reductions.
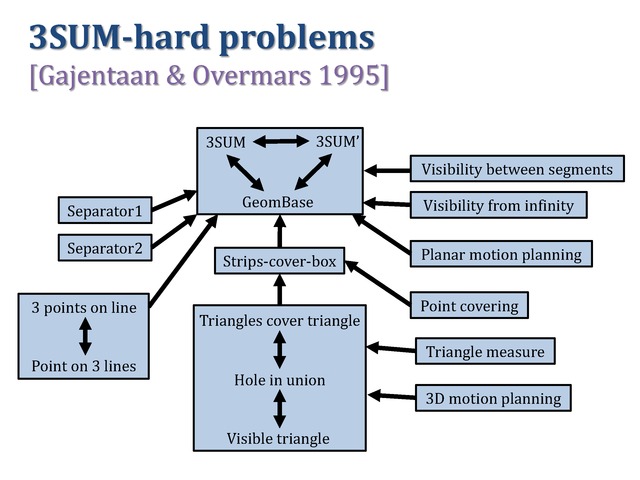
Distinct 3SUM, 3SUM′, GeomBase, 3 points on a line,
point on 3 lines, Separator, Strips cover box, Triangles cover triangle,
Hole in union, Triangle measure, Point covering, Visibility between
segments, Visible triangle, Planar motion planning, 3D motion planning,
fixed-angle chains. Nonquadratic lower bounds for triangle finding.
Conjectured cubic graph problems: diameter, all-pairs shortest paths,
negative triangle, radius, median.
|
|
This lecture is about hardness of problems that can be
solved in polynomial time but where that polynomial seems to be
substantially superlinear. Our focus will be on 3SUM (given n integers,
do any 3 sum to zero?) which is conjectured to require roughly Θ(n2)
time to solve. It has been the basis for many “3SUM-hardness” reductions,
so if any of the problems can be solved in O(n2 − ε) time, then
so can 3SUM. In class, we'll prove many problems 3SUM-hard, including
a lot of computational geometry (where 3SUM originally comes from).
We'll also talk about the generalization k-SUM, which has fairly strong
lower bounds assuming ETH, and the conjectured-cubic graph problems
all-pairs shortest paths and diameter.
|