|
[+]
3-partition I. 2-partition vs. 3-partition; variations
(Subset Sum, Numerical 3-dimensional matching, 3DM, X3C);
weakly vs. strongly NP-hard; pseudopolynomial vs. polynomial.
Multiprocessor scheduling, rectangle packing, edge-matching puzzles,
jigsaw puzzles, polyform packing puzzles,
packing squares into a square. |
|
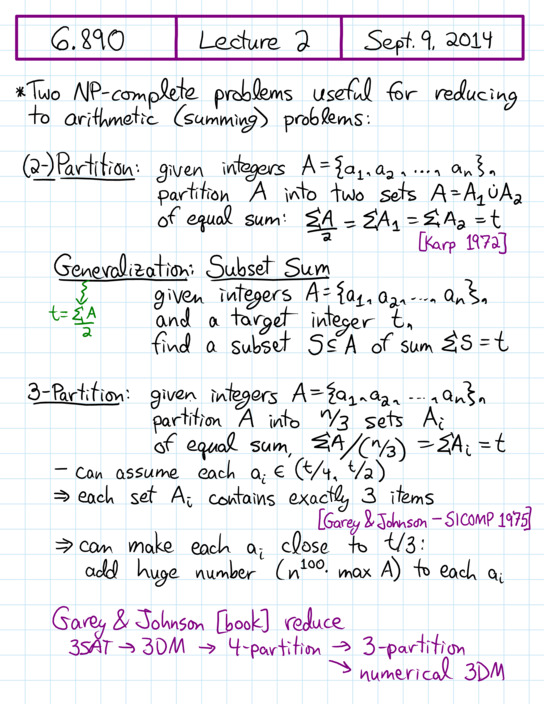
This lecture introduces my favorite (and a generally lesser known)
starting point for NP-hardness reductions, called 3-partition.
This problem is particularly useful when you have a problem that involves
adding up numbers, even when those numbers must be encoded in unary (a common
feature of many puzzles). We'll discuss many variations of the problem:
- 2-partition: Partition integers into two sets of equal sum
- Subset Sum: Select integers to equal a target sum
- 3-partition: Partition n integers into n/3 triples of equal sum
- Numerical 3-dimensional matching: Integers are of three different types,
and each triple must have all three types.
- 3-dimensional matching: A generalization to tripartite hypergraphs.
- Exact cover by 3-sets: A generalization to hypergraphs.
2-partition vs. 3-partition is an example of the weak vs. strong
NP-hardness dichotomy, and on the algorithmic side, the pseudopolynomial vs.
(weakly) polynomial dichotomy. We'll see weak and strong NP-hardness proofs,
by reductions from 2-partition and 3-partition respectively, for two problems:
- multiprocessor scheduling
- packing rectangles into a rectangle
Next we'll see a fun series
of reductions between different puzzles, starting
from 3-partition / rectangle packing to establish strong NP-hardness.
- edge-matching puzzles ("signed" like lizards, and "unsigned" like Eternity II)
- jigsaw puzzles
- polyomino packing puzzles (like Eternity)
Finally, we'll see how to prove
strong NP-hardness of
packing squares into a square. This is a handy result that we'll use
as the basis for another reduction next lecture.
|